Lecture 14: Molecular Orbital Theory

Related Resources

Lecture Notes (PDF) 

• Download English-US transcript (PDF)

The following content is provided under a Creative Commons license. Your support will help MIT OpenCourseWare continue to offer high quality educational resources for free. To make a donation or view additional materials from hundreds of MIT courses, visit MIT OpenCourseWare at ocw.mit.edu.

PROFESSOR: -- that this is not a quick clicker question, so you actually need to maybe write something down to figure out the answer. So if you haven't clicked in yet, or if you want to change your answer, keep in mind that you might need to jot down, for example, a Lewis structure before you can answer this question.

-- and get in your final answer to the clicker question. For those of you just walking in now, you might not have a chance to get all of the thought process that you need in on this clicker question, because it is based on a Lewis structure, so we will go over it. But for those of you that have been here for 30 seconds or longer, see if you can get the right answer in here.

All right, so OK, it looks like we have a very mixed response in terms of the answer to the clicker question today. Raise your hand if you didn't have time to figure out the Lewis structure. OK, so that accounts for some of you. Let's go over the correct answer this question. So, who got the right answer -- 31% of us. This is not the kind of percentages we're looking for, so let's go over this.

In class on Monday, we did go over the geometries, and the geometries themselves are very straightforward, once you know what the Lewis structure is, but remember, you can't just always look at a molecule and automatically know the Lewis structure. We actually need to think about those valence electrons. So, here we're dealing with selenium hydride, so s e h 2. I told you that there's 6 valence electrons in selenium, so we have 6 there, plus 1 for each of the hydrogen. So what we should have is 8 valence electrons in our Lewis structure. How many electrons do we need to have full valence shells?

STUDENT: [INAUDIBLE]

PROFESSOR: 12, that's right. We need 8 plus 4 is 12 for full shells. That means that we need 12 minus 8, or 4 bonding electrons in our structure. So I tried to give you one that you could draw pretty quickly, it just has hydrogens in It. So we have s e h 2. We can do our 4 bonding electrons. How many valence electrons do we have left?

STUDENT: [INAUDIBLE]

PROFESSOR: 4, that's right. So, we need to fill our octet for selenium, so 1, 2, 3, 4.

So, this is our Lewis structure here, hopefully you can see why it's not linear. If it were linear, which 32% of you seem have thought, that would have meant that our Lewis structure had no lone pairs in it, right, and that's not the case. We have two lone pairs, so if we thought about what the bonds were everywhere, it would be 109 . 5, but it's bent because we're only looking at the bonds, we're not counting the Lewis structures in naming our geometry, but they do affect the angles. And it turns out that it's actually less than 109 . 5, because those lone pairs are pushing the bonds even further away.

So, does this make sense to everyone if you think about it this way? Pretty much, okay. So, we'll try another clicker question like this later. Some of these questions that look very straightforward just naming the geometry, you have to remember to do the first step before you jump in and go ahead with a naming. So you'll got plenty of practice with this on your problem-set if you haven't already.

All right. So before we start in with today's notes, I do want to mention that this morning the Nobel Prize in chemistry was announced. This is an exciting week in science in general because we got to hear another Nobel Prize every morning pretty much. So, let's settle down for a second and start listening.

All right. So, today we're going to be talking about molecular orbital theory, but first I wanted to just mention, in case some of you didn't hear what the Nobel Prize was this morning, and this was in chemistry, it went to three different chemists. Osamu Shimomura, who's a Japanese chemist, and then Martin Chalfi who's at Columbia, and Robert Chen who's at U. C. San Diego. The three of these chemists split the Nobel Prize this morning for their discovery and/or their application of using green fluorescent protein, which is also called GFP.

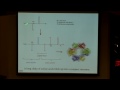
How many of you have heard of GFP before? Oh, that's so great. OK. Many of you have heard of GFP. For some of you that haven't I'll just say that it's a protein, it's 238 amino acids, which means that it's about 1,000, actually more than 1,000 atoms in size, and this protein is fluorescent. The protein was first discovered and first isolated from this jellyfish here. This was done by Shimomura, and he did this in the 60's and 70's. He actually recognized and, of course, many people recognized that this jellyfish was fluorescent, and he isolated the actual protein, and determined and proved that this protein was sufficient to cause this fluorescence.

So, in terms of thinking about applications of why it's so exciting that you have this fluorescent protein, other than it's always really fun to look at things that are fluorescent, we can think about in terms of biology why it's so exciting, you can actually tag this protein to any other protein that you're studying and now you have a visual handle on what's going on.

So, for example, if you were interested in some protein involved in cancer, you could tag it with GFP. You could watch where it localizes in the cell, there are fluorescent assays you could use to determine what other proteins it interacts with. You could see, for example, when it's expressed in a cancer cell, and where it's expressed. There are all sorts of things you can do once you can visualize something with fluorescence. The reason it is so exciting that it's a protein, and it's a protein, this is the structure here, it's a ribbon structure so you can kind of see what it looks like, it's made up of all natural amino acids. So this means we can code for it in DNA, you don't have to worry how am I going to get into the cell. All you have to do is mutate the DNA, which is very straightforward to do in molecular biology, and now you can tag absolutely any protein that you're interested in.

So, as I said, this was first discovered and isolated from the jellyfish. This was done by Shimomura -- that was kind of the first step in this process of having it become such a useful tool. And then, many years later, not until 1994 did Martin Chalfi, at Columbia, show that yes, I can, in fact, take the DNA and put it into a different organism, and he put it into e coli, a bacteria. And what he could show was that it could be expressed, this is a picture from his 1994 science paper, in that e coli, and it is going to fluoresce green. Now, the first application tends to not be quite as exciting as, for example, all the other organisms people have put it in since then -- you can have flies, you see transgenic mice that are glowing green with this GFP. Of course, that's not the useful application for it, it's more of a proof of principle, but it does show you that you can put it in for studies in any organisms.

And the field was really pushed forward by the discoveries of Robert Chen at UC San Diego, and what he did was he actually figured out how it was that this protein fluoresced, what caused the actual fluorescence. And once he did that, both he and many other scientists, could then, once they understood what caused the fluorescence, make little changes to the actual protein, and tune what the properties of that fluorescent protein were. So now, for example, there are a whole range, just a whole rainbow of fluorescent proteins that can be used. And I'm sure you can imagine that if you want to label onw protein green and one red and one yellow, now you can start looking at really complex biological processes.

So, it's pretty rare that chemistry makes the every day news. So hopefully you'll all look in the normal papers today, not just the scientific journals, and get to read something about chemistry. It's always fun to see how its described in The New York Times or in The Boston Globe.

The other thing I wanted to mention and I'm not sure if the exhibit's still there. But there was an exhibit of jellyfish, I know at least until last year, at the Boston Museum of Science, and all of you guys can get in there free. And it's neat to see the glowing jellyfish and think about the fluorescent protein that's in them. So, I encourage you to do that the next time you have some free time on your hands, maybe at IAP or some time like that.

All right, so let's move into today's notes. Today we're talking about molecular orbital theory. This is a shift, this is a new topic that we're starting. So far we've exclusively been using Lewis structures any time we've tried to describe bonding within molecules. Lewis structures are really useful, we use them all the time in chemistry. And they're useful because, first of all, they're easy to depict, they're easy to draw -- relatively easy once we get all the rules down. And also they're accurate over 90% of the time. But they're not accurate all the time in predicting bonding within molecules, and the reason for this is because Lewis structures are not, in fact, based on quantum mechanics.

So, molecular orbital theory, on the other hand, is based on quantum mechanics. And specifically, MO theory is the quantum mechanical description of wave functions within molecules. So, saying wave functions within molecules might sound a little confusing, but remember we spent a lot of time talking about wave functions within atoms, and we know how to describe that, we know that a wave function just means an atomic orbital. It's the same thing with molecules -- a molecular wave function just means a molecular orbital.

So, we'll start today talking about the two kinds of molecular orbitals, we can talk about bonding or anti-bonding orbitals. Then we're going to actually use MO theory to describe bonding within these molecules, and we'll start with homonuclear diatomic molecules. Diatomic mean it's di atomic, it's made up of two atoms, and homonuclear means that those two are the same atoms. Then at the end, we'll look at an example with a heteronuclear diatomic molecules. So, again, the same thing, but now two different atoms.

So, I will point out, in terms of MO theory, because it rigorously does take into account quantum mechanics, it starts to become complicated once we go beyond diatomic molecules. So we're going to limit in our discussion in 511-1 for molecular orbital theory to diatomic molecules. However, on Friday we will use a different approach so we can talk about bonding within atoms that have more than two atoms, molecules with more than two atoms.

All right, so one thing that I first want to point out about MO theory that is a big difference from Lewis structures, is that in MO theory valence electrons are de-localized over the entire molecule. So, when we talked about Lewis structures, we actually assigned electrons to individual atoms or to individual bonds. Whereas in molecular orbital theory, what I'm telling you is instead we understand that the electrons are spread all over the molecule, they're not just associated with a single atom or a single bond.

So specifically, what we do associate them instead is within molecular orbitals, and what we say is that they can be either in bonding or anti-bonding orbitals. And again, I want to point out that a molecular orbital, we can also call that a wave function, they're the same thing. And these orbitals arise from the combination of individual atomic orbital. So, if we have two atomic orbitals coming together from two different atoms and they combine, what we end up forming is a molecular orbital.

The reason that we can talk about this is remember that we're talking about wave functions, we're talking about waves, so we can have constructive interference in which two different orbitals can constructively interfere, we can also have destructive interference. So, we'll start by taking a look at constructive interference, and another way to explain this is just to say again, molecular orbitals are a linear combination of atomic orbitals.

So, let's start our discussion of a bonding orbital. Our simplest case that we can look at would be if we had two 1 s orbitals coming together. So let's say, for example, in a hydrogen atom. So in hydrogen atom a, I'll depict that here where the nucleus is this dot, and then the circle is what I'm depicting as the wave function. It makes sense to draw the wave function as a circle, because we do know that 1 s orbitals are spherically symmetric. So, we can say that a circle is a good approximation for a 1 s wave function.

Similarly, with the second hydrogen atom, we've got the nucleus in the middle, and the 1 s b wave function around it. So these are atomic orbitals. What we're going to do in forming a molecule is just bring these two orbitals close together such that now we have their nucleus, the two nuclei, at a distance apart that's equal to the bond length. And what we end up forming is a molecular orbital, because as we bring these two atomic orbitals close together, the part between them, that wave function, constructively interferes such that in our molecular orbital, we actually have a lot of wave function in between the two nuclei.

So we can go ahead and name our molecular orbital, just like we know how to name our atomic orbitals. And I'm going to name this sigma 1 s. The 1 s just comes from the fact that the molecular orbital is a combination of two 1 s atomic orbitals. And the sigma tells us something about the symmetry of this molecular orbital, specifically that it's cylindrically symmetric about the bond axis. So that is the bond axis -- it's just the axis between the two nuclei. Sometimes it's also called the internuclear axis. So any time you have two atoms bonding, the bond axis is just the axis that they're bonding along.

Another thing I want to point out about every sigma orbital that you see, and it will make more sense when we contrast it with pi orbitals later. But in sigma orbitals, you have no nodal planes along the bond axis, so if we had a nodal plane here, we'd see an area where the wave function was equal to zero. We don't see that. It will make more sense when we can show you one where it does have that area. But keep in mind sigma orbitals have no nodal planes along the bond axis.

All right. So, let's look at this in another way, sometimes it's hard to picture these waves combining. So let's think of them a little bit more by graphing the amplitude of the wave, and seeing how we can have this constructive interference. So again, if we think of a graph of the wave function, we had the wave function is at its highest amplitude when it's lined up with the nucleus, and then as we got further away from the nucleus, the amplitude of the wave function ends up tapering off until -- it never hits zero exactly, but it goes down very low. So we can draw that for 1 s a, we can also draw it for 1 s b, and what I'm saying for the molecular wave function is that we have the interference between the two, and we have a constructive interference, so we end up adding these two wave functions together.

So, we're talking about wave functions and we know that means orbitals, but this is -- probably the better way to think about is the physical interpretation of the wave function. So what is the wave function squared going to be equal to?

STUDENT: [INAUDIBLE]

PROFESSOR: Probability density, yes. Probability density of finding an electron within that molecule in some given volume.

So let's think about that instead, let's think about probability density. So if we're talking about probability density that's the wave function squared. But now we're talking not about an atomic wave function, we're talking about a molecular wave function. So to talk about it's squared, we're going to say it's sigma 1 s squared.

Oh, and actually before you skip your page in the notes, I realized I should write out for you what the addition is to start with. So, when we're combining two waves, what we have is 1 s a that we're adding together with 1 s for b, the second atom. And what we end up for our molecular wave function is sigma 1 s. So this is what we call our molecular orbital. All right, so that will now allow you to turn the page, I think, and we can take a look at the probability.

So the probability again, that's just the orbital squared, the wave function squared. So when we write that out, we just write sigma 1 s squared, or we can break it up into its individual parts, there's no reason we can't do that as well. So just to say that it's 1 s squared plus 1 s b, all of that together squared.

So if we write out every term individually, what we end up with is essentially just the probability density for the first atom, then the probability density for the second atom, and then we have this last term here, and this is what ends up being the interference term. So in this case where we're adding it together, this is going to be constructive interference. So in this case the cross term represents constructive interference between the two 1 s atomic wave functions. And this again is what we're going to call a bonding orbital.

So it can often make a lot more sense if we think about things in terms of energy. We've been discussing energy diagrams a lot in this class, it's a very good way to visualize exactly what's going on. So, let's think of the energy of interaction when we're comparing atomic orbitals to molecular bonding orbitals. And what you find is when you have a bonding orbital, the energy decreases compared to the atomic orbitals. So you can see here in this slide we have the atomic orbitals for the two hydrogen atoms, each of them have one electron in them, hydrogen has one electron in a 1 s orbital.

So, if we look at the molecular orbital, that's actually going to be lower in energy than either of the two atomic orbitals. So it's going to be favorable for the electrons instead to go to that lower energy state and be within the molecular orbital. So, let's draw in our electrons there, so we have our two electrons now in the molecular orbital.

So any time that you're drawing these molecular orbital diagrams, you want to keep in mind that the number of electrons that you have in atomic orbitals, you need to add those together and put that many electrons into your molecule. Right, we had one from each atom, so that means we need a total of two in our molecular orbital. And what you can see directly from looking at this energy level diagram, is that the molecule that we have is now more stable in the individual atoms. That makes sense because we're lower in energy, the electrons are now lower in energy. So that's the idea of a bonding molecular orbital.

Since we're talking about wave functions, since we're talking about the properties of waves, we don't only have constructive interference, we can also imagine a case where we would have destructive interference. Just like we see destructive interference with water waves or with light waves, we can also see destructive interference with orbitals. So, let's think about what that would look like.

So in this case we would have 1 s a and 1 s b, and instead we would subtract one from the other, and what we would see is that instead of having additional, more wave function in the middle here, we've actually cancelled out the wave function and we end up with a node. So we can also name this orbital, and this orbital we're going to call sigma 1 s star. So if we name this orbital, this is an anti-bonding molecular orbital. So we had bonding and now we're talking about anti-bonding. When we talk about anti-bonding, essentially we're taking 1 s a and now we're subtracting 1 s b, and what we end up with again is sigma 1 s, and the important thing to remember is to write this star here. So any time you see a star that means an anti-bonding orbital.

Again we can look at this in terms of thinking about a picture this way, in terms of drawing the wave function out on an axis. So we have 1 s a, and we're drawing this as having a positive amplitude, but since we have destructive interference we're going to draw 1 s b as having the opposite sign, so we have a plus and a minus in terms of signs. So that should make it very easy to picture that this is being cancelled out in the middle. If we overlay what the actual molecular orbital is on top of it, what you see is that in the center you end up cancelling out the wave function entirely. So this is the 1 s star, sigma 1 s star orbital, and what you have in the center here is a node, right in the center between the two nuclei.

So again if we look at this in terms of its physical interpretation or probability density, what we need to do is square the wave function. So if we square sigma 1 s star, we flip the amplitude so it's all positive now, but again we still have this node right in the middle. So if we talk about the probability density and we write that in, it's going to be sigma 1 s star squared, so now we're talking about 1 s a minus 1 s b, all of that being squared. And again, if we write out what all the terms are, we again have 1 s a squared plus 1 s b squared, but now what we're doing is we're actually subtracting the interference term. So if we're subtracting the interference term, what we have here now is destructive interference.

So let's think about the energy of interaction here. When we were talking about constructive interference, we had more electron density in between the 2 nuclei. So that lowered the energy of the molecular orbital. So, bonding orbitals are down here. But when we think about where anti-bonding orbitals should be, it should be higher in energy. It's increased compared to the atomic orbitals. So we would label our anti-bonding orbital higher in energy than our 1 s atomic orbitals.

So, let's think a little bit about what this means. First of all, again to repeat, any time we see the star, sigma 1 s star, that's an anti-bonding orbital. When we talk about that, basically what we're saying, and you can see that because of that negative interference, we actually have less electron density between the nuclei than we did when they were two separate atoms. So you can see that this is non-bonding, this is even worse than non-bonding, it's anti-bonding, because we're actually getting rid of electron density between the two nuclei. And we know that it's electron density between the nuclei that holds two atoms together in a bond.

So what I want to point out is that it creates an effect that is exactly opposite of a bond. You might have thought before we started talking about molecular orbital theory that non-bonding was the opposite of bonding, it's not, anti-bonding is the opposite of bonding, and anti-bonding is not non-bonding. We can see that if we just look at this picture here. Here is bonding, and here is non-bonding. Anti-bonding is even higher in energy than non-bonding. And the other thing to point out is that the energy that an anti-bonding orbital is raised by, is the same amount as a bonding orbital is lowered by. So any time I draw these molecular orbitals, I do my best, and I'm not always perfect, yet trying to make this energy different exactly the same for the anti-bonding orbital being raised, versus the bonding orbital being lowered. So those should be raised and lowered by the same energy.

So now let's take a look at some of -- is there a question up there?

STUDENT: Why would two atoms decide to [INAUDIBLE].

PROFESSOR: Well, they don't want to. It's a higher energy situation. So we'll start to look at molecules and we'll see if we take two atoms and we fill in our molecular orbital and it turns out that they have more anti-bonding orbitals than bonding, that's -- a diatomic molecule we'll never see.

So it helps us predict, will we see this, for example, h 2, which we're going to be about to do, we'll see is stabilized because it has more bonding than anti-bonding. So we'll predict, yes, there's a bond here. That's a really good question.

So, let's go ahead and do this and take a look at some of the actual atoms that we can think about and think about them in molecules. So our simplest case that we started talking about was molecular hydrogen. I want to finish this discussion by including the anti-bonding orbital, and this is a tip for you when you're drawing your molecular orbital diagrams, any time you draw a bonding orbital, there is also an anti-bonding orbital that exists. It might not have any electrons in it, but it still exists, so you need to draw these into your molecular orbital diagram. So I wanted to make sure you have a complete set for hydrogen in your notes.

So let's take a look at this. Hydrogen, we can first draw in our atomic electrons. So there's one electron in each hydrogen atom. And then this means we'll have a total of two electrons in our hydrogen molecule, so we can fill both of those into the sigma 1 s orbital, the bonding orbital. We don't have to put anything into the anti-bonding orbital, so that's great. What we've seen is we have a net lowering of energy of the molecule versus the individual atoms.

So let's draw the electron configuration of hydrogen, the molecule, molecular hydrogen. What you saw, what we've done a lot of is drawing the electron configurations for different atoms, we can do the same thing for a molecule. So, if we take h 2, and we want to draw the electron configuration, it's very short. All it is sigma 1 s, and then we have two electrons in it, so it's sigma 1 s squared.

So this is our electron configuration. Let's take a look at another example. Let's draw the molecular diagram for h e 2 now. So again, we can fill in our atomic orbitals here, there's going to be two electrons in each of our atomic orbitals. So now let's go ahead and fill in our molecular orbitals. We need to fill in a total of four electrons.

So we have two electrons in our bonding orbital, but because we use the same rules to fill up molecular orbitals as we do atomic orbitals, so the Pauli exclusion principle tells us we can't have more than two electrons per orbital, so we have to go up to our anti-bonding orbital here. So this means that we have two of the electrons are lowered in energy, but two are raised in energy. So would this be a stabilized molecule then?

STUDENT: [INAUDIBLE]

PROFESSOR: No. So, compared to the atoms, it should be somewhat the same energy, we shouldn't get any extra stabilization from forming the molecule. So lets go ahead and write what the electron configuration would be of h e 2. And again, we're just filling in the different orbitals, so we have sigma 1 s, that's going to be squared, and now we have sigma 1 s star squared.

So we can compare the two electron configurations, and we can actually think about -- what we figure out from them, we see that two are lowered in energy, two electrons are raised in energy, so we have no net gain or no net loss in energy for h e 2.

And there's actually a way that we can make predictions here, and what I'll tell you is molecular orbital theory predicts that h e 2 does not exist because it's not stabilized in terms of forming the molecule. The way that we can figure this out is using something called bond order, and bond order is equal to 1/2 times the number of bonding electrons, minus the number of anti-bonding electrons. And the bond order you get out will either be, for example, zero, which would mean that you have no bond, or you could have 1, a single bond, 1 . 5, a 1 and 1/2 bond, 2, a double bond, and so on.

So let's figure out the bond order for our two molecules here that we figured out the electron configuration for. So I guess we'll start with helium 2. So the bond order is going to be equal to 1/2, and then it will be 2 minus 2. So our bond order for h e 2 is going to be equal to 0. So it has a 0 bond, there's no bond in h e 2.

Let's look for hydrogen. For hydrogen our bond order is going to equal 1/2, 2 minus 0. So we would predict a bond order of 1. What kind of a bond is a bond order of 1? Yeah, we'd expect to see a single bond in hydrogen.

So what actually turns out the reality is that h e 2 does exist, but it exists as the weakest chemical bond known, and it wasn't, in fact, even found to exist until 1993, so I can assure you this is not a bond that you see very often in nature, and it is a very, very weak bond. It only has a dissociation energy of 0 . 1 kilojoules per mole. So that should make sense, because we saw no energy difference between the actual atoms and the molecules. Molecular orbital theory, even at this very basic level, allowed us to predict that no, we're not going to see a true bond here, a strong bond.

In contrast, the dissociation energy of a bond for hydrogen, and molecular hydrogen is everywhere around us, we see 432 kilojoules per mole.

All right, so we can now see a little bit of what the power of molecular orbital theory is in predicting what kind of bonds we're going to see in molecules, or whether or not we'll see this bonding occur at all. So let's look at another example, let's take lithium 2 and see what we can figure out here. In lithium 2, we have two atoms of lithium, each have three electrons in them. So now we have to include both the 1 s orbitals and also the 2 s orbitals. So any time in a molecular orbital diagram you draw in orbitals, you need to draw the corresponding molecular orbitals.

So, this means we need to have sigma 1 s, sigma 1 s star, and now sigma 2 s and sigma 2 s star. Something I'll also point out as you see these dashed line that tell you where the individual molecular orbitals are arising from, as you get to higher and higher atomic numbers of molecules that you're making, it makes a lot more sense to look at a diagram when you draw these dotted lines in, because they can start to get a little bit confusing. So when you go ahead and draw these on your problem-sets or on your exams, it's a good idea to put these dashed lines in, both for you and for people reading it to see exactly where your molecular orbitals are coming from.

So, this means we have a total of six electrons that we need to put into molecular orbitals. So again we just start filling those up -- we have two in the 1 s, two in the sigma 1 s star, and then we have two in the sigma 2 s. So we should be able to also calculate the bond order, just like we did for hydrogen and helium. First we can do that by knowing the electron configuration, we can write it out just by going up the table here, up the energy levels. And you can go ahead and tell me what you think the bond order is going to be for this molecule.

All right. Let's take 10 more seconds on this. OK, good, we're back on track a little bit with our clicker answers. So it's selection three or one is the bond order. So let's switch back to our class notes and look at what this means. So we know that it's 1, because we have 1, 2, 3, 4 bonding, minus 2 anti-bonding, and 1/2 of that is a bond order of 1. We would predict to see a single bond between lithium, and it turns out that's what we see. And so you have for a reference, the dissociation energy of lithium 2 is 105 kilojoules per mole.

So let's keep moving along the periodic table and keep applying our molecular orbitals. For example now, with b e 2, so beryllium 2 has four electrons in terms of each atom. So you can start by filling those in, and now we can fill in our molecular orbitals as well. This means we need a total of eight electrons in our molecular orbitals. So we have two in 1 s, two in the sigma 1 s star, two in the sigma 2 s, and two in the sigma 2 s star.

So let's go ahead and figure out the bonding order for beryllium here. So when we figure it out for beryllium -- let's see if I wrote in your notes what the actual -- electron configuration, OK that's already in your notes for you. So let's go right to the bond order for beryllium. So for the bond order we want to take 1/2 of the total number of bonding electrons, so that's going to be 4 minus anti-bonding is 4, so we end up getting a bond order that's equal to 0.

So what I want to point out with this case in beryllium is that you don't have to use all of the electrons to figure out the bond order, and in fact, once you get to molecules that are from atoms with atomic numbers of 8 or 10, you're not going to want to maybe draw out the full molecular orbital diagram. So what I want to tell you is we also always get the same bond order if we instead only deal with the valence electrons. So let's just prove that to ourselves and figure out the bond order just using valence electrons. So this would mean the bond order is equal to 1/2, and in terms of valence electrons, how many bonding valence electrons do we have?

STUDENT: [INAUDIBLE]

PROFESSOR: All right, what about anti-bonding?

STUDENT: [INAUDIBLE]

PROFESSOR: Two. OK, good. So again, we're going to see that we have a bonding order of 0. So we would not predict to see a b e 2 bond. So what we see is a bond order of 0, and again, the bond is very, very weak. Essentially we're not going to see this, it's 9 kilojoules per mole.

All right. So, so far we've looked only at molecules that involve atoms that have only s orbitals in them. I'm sure you're thinking well, what do we do in the case of p orbitals, and, in fact, we can do the same thing. Again, we're going to take the linear combination of those p atomic orbitals and make what are called pi or some more sigma molecular orbitals.

So let's look at the first case where we have either the 2 p x or 2 p y type of orbitals that we're combining. So they're the same shape, this is the shape of the orbital or the shape of the wave function, and we can call this either 2 p x a being combined with 2 p x b, or we could say since it's the same shape, it's 2 p y a being combined with 2 p y b. And in either case if we first talk about constructive interference, what again we're going to see is that where these two orbitals come together, we're going to see increased wave function in that area, so we saw constructive interference.

So again, we can name these molecular orbitals and these we're going to call -- also to point out there is now a bond axis along this nodal plane, which is something we didn't see before when we were combining the s orbitals. So when we go ahead and name these, we're going to call these pi orbitals. We'll call it either pi 2 p x, if we're combining the x orbitals, or pi 2 p y.

The reason that I wanted to point out this nodal plane here is because this is why it is called a pi orbital. Pi orbitals are a molecular orbital that have a nodal plane through the bond axis. Remember this is our bond axis here, and you can see there is this area where the wave function is equal to zero all along that plane, that's a nodal plane. So that's why these are pi orbitals instead of sigma orbitals.

So again, we can think about the probability density in terms of squaring the wave function. So now what it is that we're squaring is if we're talking about x orbital, it's pi 2 p x squared, and this is just equal to the 2 p x a plus the 2 p x b all squared, or if we write out all of the terms we have 2 p x a squared plus 2 p x b squared, and then this term here, and again, this is our interference term. In this case is it constructive or destructive interference?

STUDENT: [INAUDIBLE]

PROFESSOR: Constructive interference. We're seeing that the wave function's adding together and giving us more wave function in the center here.

All right. So we see constructive interference, of course, we can also see destructive interference. So I changed the colors here to show that these are 2 p orbitals with an opposite phase or an opposite sign. So what happens when we add a 2 p a and we subtract from it a 2 p x b, or the same with a 2 p y a subtracting a 2 p y b, is that we're actually going to cancel out the wave function in the center, so we now have 2 nodal planes. So again, this is an anti-bonding orbital, and what you see is that there is now less electron density between the two nuclei than there was when you had non-bonding.

So we're going to call this the sigma 2 p x star, or if we're talking about the 2 p y orbitals we'll call this the pi 2 p x star, and the pi 2 p y star. And the pi star orbitals result from any time you have destructive interference from 2 p orbitals that are either the p x or the p y.

So now we can move on to an example where we do, in fact, have to use some p orbitals, so this would be b 2. How many electrons are in boron? Five. I see some hands going up. There's five electrons So what you'll notice here is that I only filled in 3 electrons. What do these electrons represent?

STUDENT: Valence.

PROFESSOR: Valence electrons. OK, sometimes you're going to be asked to draw a molecular orbital diagram where you're asked to include all electrons, and sometimes it will specifically say only include valence electrons. That happens because of space issues that you were asked to do that, because you can always assume that all of the core orbitals are already going to be filled. So, in this case, we're just drawing the molecular orbital diagram for the valence electrons, so we have three for each. And what we see here is now when we're combining the p, we have our 2 p x and our 2 p y orbitals that are lower in energy, and then our pi anti-bonding orbitals that are higher in energy. You might be asking where the 2 p z orbital is and we'll get to that soon once we need it. Let's just first fill in this for the b 2 case. So we can start at the bottom, two electrons in sigma 2 s, two electrons in sigma 2 s star. Now we need to jump up to using these pi orbitals, and what we're going to do is put one electron into each of our pi 2 p x and 2 p y orbitals.

So again you can see as we're filling up our molecular orbitals, we're using the exact same principle we used to fill up atomic orbitals.

So let's think about what the valence electron configuration is here. So now we're looking at the case of b 2. And what we're looking at is a valence electron molecular orbital diagram, so let's just draw the electron configuration for the valence orbitals, so that will be sigma 2 s 2, sigma 2 s star 2, and now we start in with our pi 2 p x 1, and our pi 2 p y 1. So this is our valence electron configuration for b 2.

All right. So what would you expect the bonding order for b 2 to be? Shout it out if you know. STUDENT: one.

PROFESSOR: And I didn't write up there but it is one, and we can see that it's 1, because it's 1/2 of 2, 4 minus 2, so 1/2 of 2, the bonding order is going to be equal to one.

So let's move on to another example, let's talk about carbon here. Again, we're just talking about the valence electrons. So carbon has four valence electrons, so if we talk about c 2, again we're going to start filling in our molecular orbitals, and now we're going to have eight electrons to fill into our molecular orbitals. So, we'll put two in the sigma 2 s, two in the sigma 2 s star, and now we're going to fill one and one into each of our pi 2 p x and 2 p y, but we still have two electrons left, so what we're going to do is double up in terms of our 2 p x and our 2 p y.

So let's think about what this valence electron configuration is for c 2. And again, I want you to have practiced drawing these out in the form -- you always need to start with the sigma and then write the number of the orbital. So, sigma 2 s has two electrons, sigma 2 s star with two electrons, and now we have sigma 2 p x -- how many electrons here?

STUDENT: Two.

PROFESSOR: Two. And sigma 2 p y, two electrons here. Oh excuse me, pi 2 p y, thank you -- pi 2 p x and pi 2 p y. So let's talk about what the bonding order is going to be for c 2. So what's the bonding order for c 2?

STUDENT: Two.

PROFESSOR: two? OK, so we have 2, 4, 6 minus 2, so we have 1/2 of 6 minus 2, so that's 1/2 half 4, so we have a bonding order of two for carbon 2. So we would expect to see a double bond for a c 2 where we would expect to see a -- double bond for c 2 and a single bond for b 2.

And that is, in fact, what we can surmise if we look at the different dissociation energies for the two bonds. So for b 2, which is a single bond, that's 289 kilojoules per mole to break it, and it takes us more energy to break this double bond for carbon, which is 599 kilojoules per mole.

So, in general what we see, and this is always true if we're comparing the same atom, and in general, if we're comparing different types of molecules, but we know that a single bond is always weaker than a double bond, which is weaker than a triple bond. And obviously, no bond is the weakest of all is not bonding.

All right. So let's look now at the case where we do have 2 p z orbitals that we're talking about. So again, what we're talking about is the linear combination of atomic 2 p orbitals, and now we're talking about 2 p z. So if we have constructive interference between the two, what we're going to see is our molecular orbital looks something like this. Do you predict that this will be a sigma or a pi orbital?

STUDENT: [INAUDIBLE]

PROFESSOR: All right, I'm hearing a little of both, but I'm very encouraged to hear quite a few people saying sigma. This is, in fact, a sigma 2 p z orbital is what this orbital is called. The reason that it's sigma is if you look at the bonding axis here, is that there is no nodal plane along the bonding axis. Also, it is cylindrically symmetric around the bonding axis, so this is how we know that it's a sigma orbital. So some p orbitals form pi molecular orbitals, and some form sigma p orbitals. Specifically, it's always the z that forms the sigma orbital, and the reason is at least at a minimum for this class we always define the internuclear axis as the z axis, so this is always the z axis, so it's always going to be the 2 p z's that are coming together head-on. The reason that there is increased electron density here is you can see that these two orbitals come together and constructively interfere.

We can also talk about anti-bonding orbitals where we have destructive interference. So instead, these would be canceling out wave functions between the two, so we would end up with a nodal plane down the center. Would this be a sigma or a pi? It's still sigma. So even though we see a nodal plane down the center, I just want to really point out that it's only when we have a nodal plane in the internuclear or the bond axis that we're calling that a pi orbital. So this is still a sigma -- it's a sigma 2 p z star orbital. We have destructive interference here.

So now let's look at an example where we talk about using these 2 p z orbitals, so let's look at oxygen. So the first thing I want to point out is that the 2 p z, the sigma 2 p z is even lower in energy than the pi orbitals here, and the anti-bonding sigma orbital is going to be higher in energy than the pi 2 p orbitals that we have here. So in oxygen again, this is just showing the valence electrons, so we end up having six valence electrons from each oxygen atom. We can fill right up our table just like we did before, but now we have included our 2 p z orbital here.

So we have two electrons in sigma 2 s, two in sigma 2 s star, in sigma 2 p z we have two -- those are filled first, and now we're going to put one into pi 2 p x, and one into pi 2 p y.

So we can write out what the electron configuration is here, and I think that I have already written that out for you in your notes. What is the bond order of o 2? It's two. Oh excuse me, I didn't fill in all of my electrons. All right. So we had a total of 2, 4, 6, 8. So we had a total of 12. So we filled in four here -- we need to keep going. All right, so I did this not at all purposely, but this can point out for you that you need to make sure that the number of electrons that you have in your molecular orbital does match up with the total number that you have in your atomic orbitals. So I did not do any counting up here, you should make sure you do counting, I apologize. So we need to fill all the way up to the pi 2 p x, and the pi 2 p y.

All right, so the bonding order, you're correct, should be 2, if we subtract the number of bonding minus anti-bonding electrons and take that in 1/2. And what I want to point out that we just figured out for molecular orbital theory, is that o 2 is a biradical, because remember, the definition of a radical is when we have an unpaired electron. You can see that we have two unpaired electrons in this molecule here -- one in the pi 2 p x star, and one in the pi 2 p y star orbital. This was something we could not predict using Lewis structures, but we can predict using MO theory that we have a radical species here. So that's a really important type of an application that we can use MO theory for that we weren't able to do with our Lewis structures.

All right, I want to do one more at homonuclear example here, and this is n 2. The first thing that I need to point out is you can actually see an n 2 versus o 2 that we flip-flopped the energy of the sigma and the pi 2 p orbitals. So this is a glitch, just like sometimes we had glitches in filling up our atomic orbitals. This is something that you need to remember. And what you need to remember is if the z is equal to eight or greater, such as oxygen being the cut-off point, this sigma 2 p orbital is actually lower in energy than the pi 2 p orbitals, the molecular orbitals. But for anything 7 or less, so what is the atomic number for nitrogen?

STUDENT: Five.

PROFESSOR: five -- there's five valence electrons, but the atomic number is actually seven. So z equals 7 -- this is the cut-off where, in fact, the sigma orbital is going to be higher in energy than the pi 2 p orbitals. This is something that you just need to remember. I wrote it down your notes, if you can put a big star next to it so you don't forget this. This is something you're going to be responsible for in drawing out your molecular orbitals. So let's fill it out in this way, keeping in mind that we're going to fill out the pi 2 p's before the sigma. So we have a total of 2, 4, 6, 8, 10 valence electrons, so I'll make sure I count to 10 as we fill up our molecular orbitals here. We have two, then we have four. Now we're going to start in with that pi 2 p orbitals, which gives us 1 each, and then two each in those, and then after that, we'll go up to our sigma 2 p z orbital.

So this is going to be our molecular orbital diagram. And again, I've written for you, but you can figure out what the electron configuration is just by writing up in this order here. And what is the bond order going to be n 2?

STUDENT: [INAUDIBLE]

PROFESSOR: It's going to be three. So, you can go ahead and calculate that, if you can't see that right away.

So we'll end here, we'll finish up with the heteronuclear example on Friday.